Formule de Taylor-Young : ![]() . Alors
. Alors ![]() admet un développement limité au voisinage de
admet un développement limité au voisinage de ![]() :
:
![Rendered by QuickLaTeX.com \[ f(x) \underset{a}{=} \sum_{k=0}^n \dfrac{f^{(k)}a}{k!} (x-a)^k + o((x-a)^n) \]](http://www.rozenblum.com/wp-content/ql-cache/quicklatex.com-b438c172d79d2f222d7e0d1498cfbe05_l3.png)
DÉVELOPPEMENTS LIMITÉS USUELS EN ZÉRO
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
Ce qui est affirmé sans preuve peut être nié sans preuve (Euclide). Le but ultime n'est rien, le mouvement est tout (Eduard Bernstein)
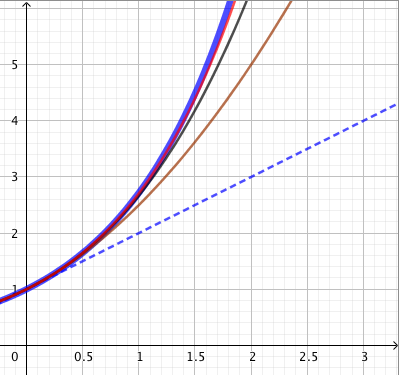
Formule de Taylor-Young : ![]() . Alors
. Alors ![]() admet un développement limité au voisinage de
admet un développement limité au voisinage de ![]() :
:
![Rendered by QuickLaTeX.com \[ f(x) \underset{a}{=} \sum_{k=0}^n \dfrac{f^{(k)}a}{k!} (x-a)^k + o((x-a)^n) \]](http://www.rozenblum.com/wp-content/ql-cache/quicklatex.com-b438c172d79d2f222d7e0d1498cfbe05_l3.png)
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()